What Is Domain and Range Again

Functions: Domain and Range

Permit's render to the subject of domains and ranges.

When functions are starting time introduced, you will probably have some simplistic "functions" and relations to bargain with, usually being just sets of points. These won't exist terribly useful or interesting functions and relations, but your text wants you to get the thought of what the domain and range of a function are. Small sets of points are generally the simplest sorts of relations, then your volume starts with those.

For case:

Content Continues Below

• State the domain and range of the following relation. Is the relation a function?

{(2, –3), (four, 6), (3, –ane), (6, 6), (2, 3)}

The in a higher place list of points, being a relationship between certain x 's and certain y 's, is a relation. The domain is all the x -values, and the range is all the y -values. To requite the domain and the range, I just list the values without duplication:

domain: {2, three, four, 6}

range: {–3, –1, 3, 6}

(It is customary to list these values in numerical order, but it is non required. Sets are chosen "unordered lists", so you can list the numbers in any gild you feel like. Just don't duplicate: technically, repetitions are okay in sets, merely near instructors would count off for this.)

While the given set does indeed stand for a relation (because x 'southward and y 'southward are being related to each other), the set they gave me contains two points with the aforementioned x -value: (two, –3) and (2, 3). Since 10 = 2 gives me two possible destinations (that is, 2 possible y -values), and then this relation is not a role.

Note that all I had to do to check whether the relation was a function was to look for duplicate 10 -values. If you find any indistinguishable 10 -values, so the different y -values hateful that y'all do not take a function. Retrieve: For a relation to be a function, each x-value has to go to one, and only one, y-value.

• State the domain and range of the post-obit relation. Is the relation a role?

{(–3, 5), (–2, five), (–1, 5), (0, five), (1, 5), (2, 5)}

I'll just listing the 10 -values for the domain and the y -values for the range:

domain: {–three, –two, –i, 0, one, 2}

range: {v}

This is another instance of a "boring" function, simply similar the example on the previous folio: every concluding x -value goes to the exact same y -value. But each x -value is different, so, while tiresome,

this relation is indeed a function.

In point of fact, these points lie on the horizontal line y = 5.

---

By the way, the name for a set with only one element in it, like the "range" set up higher up, is "singleton". And so the range could besides be stated as "the singleton of v"

---

There is i other case for finding the domain and range of functions. They will give you a role and ask you to find the domain (and mayhap the range, too). I have only e'er seen (or can even think of) 2 things at this stage in your mathematical career that you lot'll have to bank check in club to determine the domain of the function they'll requite you, and those two things are denominators and foursquare roots.

• Make up one's mind the domain and range of the given office:

The domain is all the values that 10 is allowed to have on. The only problem I take with this function is that I need to be conscientious not to divide past zero. And so the only values that 10 tin can not have on are those which would cause division by aught. Then I'll set the denominator equal to null and solve; my domain will be everything else.

ten ⁱⁱ – ten – 2 = 0

(x – 2)(10 + 1) = 0

x = 2 or 10 = –1

And so the domain is "all x not equal to –1 or 2".

The range is a bit trickier, which is why they may not ask for information technology. In full general, though, they'll want you to graph the function and find the range from the moving picture. In this case:

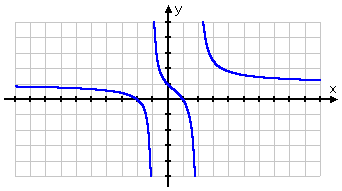
Equally you lot tin can encounter from my motion-picture show, the graph "covers" all y -values; that is, the graph will go equally low as I like, and will also go as high as I like. For whatever bespeak on the y -centrality, no matter how high up or low down, I can go from that betoken either to the right or to the left and, somewhen, I'll cross the graph. Since the graph will eventually cover all possible values of y , then:

the range is "all existent numbers".

• Determine the domain and range of the given function:

The domain is all values that x can take on. The only trouble I have with this function is that I cannot accept a negative within the square root. So I'll gear up the insides greater-than-or-equal-to zero, and solve. The result volition be my domain:

–210 + 3 ≥ 0

–2ten ≥ –three

twox ≤ three

x ≤ 3/two = ane.5

Then the domain is "all ten ≤ iii/two".

The range requires a graph. I need to exist careful when graphing radicals:

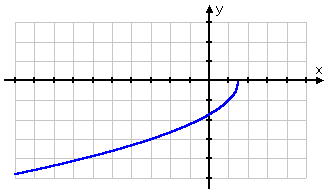
The graph starts at y = 0 and goes downwardly (heading to the left) from there. While the graph goes down very slowly, I know that, eventually, I can go equally depression as I similar (past picking an 10 that is sufficiently big). Also, from my feel with graphing, I know that the graph volition never starting time coming back up. Then:

the range is "all y ≤ 0".

• Determine the domain and range of the given function:

y = –x ⁴ + 4

This is simply a garden-variety polynomial. There are no denominators (and then no partitioning-by-zippo issues) and no radicals (and then no square-root-of-a-negative issues). There are no problems with a polynomial. There are no values that I tin can't plug in for x . When I have a polynomial, the answer for the domain is always:

the domain is "all ten ".

The range will vary from polynomial to polynomial, and they probably won't even ask, but when they do, I look at the picture:

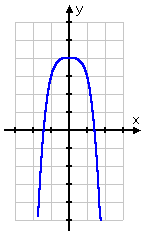
The graph goes only as high as y = 4, simply it will go as low equally I like. And so:

The range is "all y ≤ 4".

spanoofue1966.blogspot.com

Source: https://www.purplemath.com/modules/fcns2.htm

Share this post